Rubber bracelets

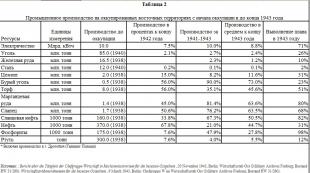
Vieta's theorem. Examples of using. How to solve equations using Vieta's theorem in mathematics Vieta's formula for an equation

In mathematics, there are special tricks with which many quadratic equations are solved very quickly and without any discriminants. Moreover, with proper training, many begin to solve quadratic equations verbally, literally "at a glance."

Unfortunately, in the modern course of school mathematics, such technologies are almost not studied. And you need to know! And today we will consider one of these techniques - Vieta's theorem. First, let's introduce a new definition.

A quadratic equation of the form x 2 + bx + c = 0 is called reduced. Please note that the coefficient at x 2 is equal to 1. There are no other restrictions on the coefficients.

x 2 + 7x + 12 = 0 is the reduced quadratic equation;
x 2 − 5x + 6 = 0 is also reduced;
2x 2 − 6x + 8 = 0 - but this is not given at all, since the coefficient at x 2 is 2.

Of course, any quadratic equation of the form ax 2 + bx + c = 0 can be made reduced - it is enough to divide all the coefficients by the number a . We can always do this, since it follows from the definition of a quadratic equation that a ≠ 0.

True, these transformations will not always be useful for finding roots. A little lower, we will make sure that this should be done only when in the final squared equation all the coefficients are integer. For now, let's look at some simple examples:

A task. Convert quadratic equation to reduced:

3x2 − 12x + 18 = 0;

−4x2 + 32x + 16 = 0;

1.5x2 + 7.5x + 3 = 0;

2x2 + 7x − 11 = 0.

Let's divide each equation by the coefficient of the variable x 2 . We get:

3x 2 - 12x + 18 \u003d 0 ⇒ x 2 - 4x + 6 \u003d 0 - divided everything by 3;
−4x 2 + 32x + 16 = 0 ⇒ x 2 − 8x − 4 = 0 - divided by −4;
1.5x 2 + 7.5x + 3 \u003d 0 ⇒ x 2 + 5x + 2 \u003d 0 - divided by 1.5, all coefficients became integer;
2x 2 + 7x - 11 \u003d 0 ⇒ x 2 + 3.5x - 5.5 \u003d 0 - divided by 2. In this case, fractional coefficients arose.

As you can see, the given quadratic equations can have integer coefficients even if the original equation contained fractions.

Now we formulate the main theorem, for which, in fact, the concept of a reduced quadratic equation was introduced:

Vieta's theorem. Consider the reduced quadratic equation of the form x 2 + bx + c \u003d 0. Suppose that this equation has real roots x 1 and x 2. In this case, the following statements are true:

x1 + x2 = −b. In other words, the sum of the roots of the given quadratic equation is equal to the coefficient of the variable x, taken with the opposite sign;

x 1 x 2 = c. The product of the roots of a quadratic equation is equal to the free coefficient.

Examples. For simplicity, we will consider only the given quadratic equations that do not require additional transformations:

x 2 − 9x + 20 = 0 ⇒ x 1 + x 2 = − (−9) = 9; x 1 x 2 = 20; roots: x 1 = 4; x 2 \u003d 5;
x 2 + 2x − 15 = 0 ⇒ x 1 + x 2 = −2; x 1 x 2 \u003d -15; roots: x 1 = 3; x 2 \u003d -5;
x 2 + 5x + 4 = 0 ⇒ x 1 + x 2 = −5; x 1 x 2 = 4; roots: x 1 \u003d -1; x 2 \u003d -4.

Vieta's theorem gives us additional information about the roots of a quadratic equation. At first glance, this may seem complicated, but even with minimal training, you will learn to "see" the roots and literally guess them in a matter of seconds.

A task. Solve the quadratic equation:

x2 − 9x + 14 = 0;

x 2 - 12x + 27 = 0;

3x2 + 33x + 30 = 0;

−7x2 + 77x − 210 = 0.

Let's try to write down the coefficients according to the Vieta theorem and "guess" the roots:

x 2 − 9x + 14 = 0 is a reduced quadratic equation.
By the Vieta theorem, we have: x 1 + x 2 = −(−9) = 9; x 1 x 2 = 14. It is easy to see that the roots are the numbers 2 and 7;
x 2 − 12x + 27 = 0 is also reduced.
By the Vieta theorem: x 1 + x 2 = −(−12) = 12; x 1 x 2 = 27. Hence the roots: 3 and 9;
3x 2 + 33x + 30 = 0 - This equation is not reduced. But we will fix this now by dividing both sides of the equation by the coefficient a \u003d 3. We get: x 2 + 11x + 10 \u003d 0.
We solve according to the Vieta theorem: x 1 + x 2 = −11; x 1 x 2 = 10 ⇒ roots: −10 and −1;
−7x 2 + 77x − 210 \u003d 0 - again the coefficient at x 2 is not equal to 1, i.e. equation not given. We divide everything by the number a = −7. We get: x 2 - 11x + 30 = 0.
By the Vieta theorem: x 1 + x 2 = −(−11) = 11; x 1 x 2 = 30; from these equations it is easy to guess the roots: 5 and 6.

From the above reasoning, it can be seen how Vieta's theorem simplifies the solution of quadratic equations. No complicated calculations, no arithmetic roots and fractions. And even the discriminant (see the lesson " Solving quadratic equations") We did not need.

Of course, in all our reflections, we proceeded from two important assumptions, which, generally speaking, are not always fulfilled in real problems:

The quadratic equation is reduced, i.e. the coefficient at x 2 is 1;
The equation has two different roots. From the point of view of algebra, in this case the discriminant D > 0 - in fact, we initially assume that this inequality is true.

However, in typical mathematical problems these conditions are met. If the result of the calculations is a “bad” quadratic equation (the coefficient at x 2 is different from 1), this is easy to fix - take a look at the examples at the very beginning of the lesson. I am generally silent about the roots: what kind of task is this in which there is no answer? Of course there will be roots.

Thus, the general scheme for solving quadratic equations according to the Vieta theorem is as follows:

Reduce the quadratic equation to the given one, if this has not already been done in the condition of the problem;
If the coefficients in the above quadratic equation turned out to be fractional, we solve through the discriminant. You can even go back to the original equation to work with more "convenient" numbers;
In the case of integer coefficients, we solve the equation using the Vieta theorem;
If within a few seconds it was not possible to guess the roots, we score on the Vieta theorem and solve through the discriminant.

A task. Solve the equation: 5x 2 − 35x + 50 = 0.

So, we have an equation that is not reduced, because coefficient a \u003d 5. Divide everything by 5, we get: x 2 - 7x + 10 \u003d 0.

All coefficients of the quadratic equation are integer - let's try to solve it using Vieta's theorem. We have: x 1 + x 2 = −(−7) = 7; x 1 x 2 \u003d 10. In this case, the roots are easy to guess - these are 2 and 5. You do not need to count through the discriminant.

A task. Solve the equation: -5x 2 + 8x - 2.4 = 0.

We look: −5x 2 + 8x − 2.4 = 0 - this equation is not reduced, we divide both sides by the coefficient a = −5. We get: x 2 - 1.6x + 0.48 \u003d 0 - an equation with fractional coefficients.

It is better to return to the original equation and count through the discriminant: −5x 2 + 8x − 2.4 = 0 ⇒ D = 8 2 − 4 (−5) (−2.4) = 16 ⇒ ... ⇒ x 1 = 1.2; x 2 \u003d 0.4.

A task. Solve the equation: 2x 2 + 10x − 600 = 0.

To begin with, we divide everything by the coefficient a \u003d 2. We get the equation x 2 + 5x - 300 \u003d 0.

This is the reduced equation, according to the Vieta theorem we have: x 1 + x 2 = −5; x 1 x 2 \u003d -300. It is difficult to guess the roots of the quadratic equation in this case - personally, I seriously "froze" when I solved this problem.

We will have to look for roots through the discriminant: D = 5 2 − 4 1 (−300) = 1225 = 35 2 . If you don't remember the root of the discriminant, I'll just note that 1225: 25 = 49. Therefore, 1225 = 25 49 = 5 2 7 2 = 35 2 .

Now that the root of the discriminant is known, solving the equation is not difficult. We get: x 1 \u003d 15; x 2 \u003d -20.

When studying ways to solve second-order equations in a school algebra course, consider the properties of the roots obtained. They are now known as Vieta's theorems. Examples of its use are given in this article.

Quadratic equation

The second order equation is an equality, which is shown in the photo below.

Here the symbols a, b, c are some numbers that are called the coefficients of the equation under consideration. To solve an equality, you need to find x values that make it true.

Note that since the maximum value of the power to which x is raised is two, then the number of roots in the general case is also two.

There are several ways to solve this type of equality. In this article, we will consider one of them, which involves the use of the so-called Vieta theorem.

Statement of Vieta's theorem

At the end of the 16th century, the famous mathematician Francois Viet (Frenchman) noticed, analyzing the properties of the roots of various quadratic equations, that certain combinations of them satisfy specific relationships. In particular, these combinations are their product and sum.

Vieta's theorem establishes the following: the roots of a quadratic equation, when summed, give the ratio of the linear to quadratic coefficients taken with the opposite sign, and when they are multiplied, they lead to the ratio of the free term to the quadratic coefficient.

If the general form of the equation is written as it is shown in the photo in the previous section of the article, then mathematically this theorem can be written as two equalities:

r 2 + r 1 \u003d -b / a;
r 1 x r 2 \u003d c / a.

Where r 1 , r 2 is the value of the roots of the considered equation.

These two equalities can be used to solve a number of very different mathematical problems. The use of the Vieta theorem in examples with a solution is given in the following sections of the article.

Between the roots and the coefficients of the quadratic equation, in addition to the root formulas, there are other useful relationships that are given by Vieta's theorem. In this article, we will give a formulation and proof of Vieta's theorem for a quadratic equation. Next, we consider a theorem converse to Vieta's theorem. After that, we will analyze the solutions of the most characteristic examples. Finally, we write down the Vieta formulas that define the connection between the real roots algebraic equation degree n and its coefficients.

Page navigation.

Vieta's theorem, formulation, proof

From the formulas of the roots of the quadratic equation a x 2 +b x+c=0 of the form , where D=b 2 −4 a c , the relations x 1 +x 2 = −b/a, x 1 x 2 = c/a . These results are confirmed Vieta's theorem:

Theorem.

If a x 1 and x 2 are the roots of the quadratic equation a x 2 +b x+c=0, then the sum of the roots is equal to the ratio of the coefficients b and a, taken with the opposite sign, and the product of the roots is equal to the ratio of the coefficients c and a, that is, .

Proof.

We will prove the Vieta theorem according to the following scheme: we will compose the sum and product of the roots of the quadratic equation using the known root formulas, then we will transform the resulting expressions, and make sure that they are equal to −b/a and c/a, respectively.

Let's start with the sum of the roots, compose it. Now we bring the fractions to a common denominator, we have. In the numerator of the resulting fraction , after which : . Finally, after 2 , we get . This proves the first relation of the Vieta theorem for the sum of the roots of a quadratic equation. Let's move on to the second.

We compose the product of the roots of the quadratic equation:. According to the rule of multiplication of fractions, the last product can be written as. Now we multiply the bracket by the bracket in the numerator, but it is faster to collapse this product by difference of squares formula, So . Then, remembering , we perform the next transition . And since the formula D=b 2 −4 a·c corresponds to the discriminant of the quadratic equation, then b 2 −4·a·c can be substituted into the last fraction instead of D, we get . After opening the brackets and reducing like terms, we arrive at the fraction , and its reduction by 4·a gives . This proves the second relation of Vieta's theorem for the product of roots.

If we omit the explanations, then the proof of the Vieta theorem will take a concise form:
,
.

It remains only to note that when the discriminant is equal to zero, the quadratic equation has one root. However, if we assume that the equation in this case has two identical roots, then the equalities from the Vieta theorem also hold. Indeed, for D=0 the root of the quadratic equation is , then and , and since D=0 , that is, b 2 −4·a·c=0 , whence b 2 =4·a·c , then .

In practice, Vieta's theorem is most often used in relation to the reduced quadratic equation (with the highest coefficient a equal to 1 ) of the form x 2 +p·x+q=0 . Sometimes it is formulated for quadratic equations of just this type, which does not limit the generality, since any quadratic equation can be replaced by an equivalent equation by dividing both its parts by a non-zero number a. Here is the corresponding formulation of Vieta's theorem:

Theorem.

The sum of the roots of the reduced quadratic equation x 2 + p x + q \u003d 0 is equal to the coefficient at x, taken with the opposite sign, and the product of the roots is a free term, that is, x 1 + x 2 \u003d −p, x 1 x 2 \u003d q .

Theorem inverse to Vieta's theorem

The second formulation of the Vieta theorem, given in the previous paragraph, indicates that if x 1 and x 2 are the roots of the reduced quadratic equation x 2 +p x+q=0, then the relations x 1 +x 2 = −p, x 1 x 2=q. On the other hand, from the written relations x 1 +x 2 =−p, x 1 x 2 =q, it follows that x 1 and x 2 are the roots of the quadratic equation x 2 +p x+q=0. In other words, the assertion converse to Vieta's theorem is true. We formulate it in the form of a theorem, and prove it.

Theorem.

If the numbers x 1 and x 2 are such that x 1 +x 2 =−p and x 1 x 2 =q, then x 1 and x 2 are the roots of the reduced quadratic equation x 2 +p x+q=0.

Proof.

After replacing the coefficients p and q in the equation x 2 +p x+q=0 of their expression through x 1 and x 2, it is converted into an equivalent equation.

We substitute the number x 1 instead of x into the resulting equation, we have the equality x 1 2 −(x 1 + x 2) x 1 + x 1 x 2 =0, which for any x 1 and x 2 is the correct numerical equality 0=0, since x 1 2 −(x 1 + x 2) x 1 + x 1 x 2 = x 1 2 −x 1 2 −x 2 x 1 + x 1 x 2 =0. Therefore, x 1 is the root of the equation x 2 −(x 1 + x 2) x + x 1 x 2 \u003d 0, which means that x 1 is the root of the equivalent equation x 2 +p x+q=0 .

If in the equation x 2 −(x 1 + x 2) x + x 1 x 2 \u003d 0 substitute the number x 2 instead of x, then we get the equality x 2 2 −(x 1 + x 2) x 2 + x 1 x 2 =0. This is the correct equation because x 2 2 −(x 1 + x 2) x 2 + x 1 x 2 = x 2 2 −x 1 x 2 −x 2 2 +x 1 x 2 =0. Therefore, x 2 is also the root of the equation x 2 −(x 1 + x 2) x + x 1 x 2 \u003d 0, and hence the equations x 2 +p x+q=0 .

This completes the proof of the theorem converse to Vieta's theorem.

Examples of using Vieta's theorem

It's time to talk about the practical application of Vieta's theorem and its inverse theorem. In this subsection, we will analyze the solutions of several of the most typical examples.

We start by applying a theorem converse to Vieta's theorem. It is convenient to use it to check whether the given two numbers are the roots of a given quadratic equation. In this case, their sum and difference are calculated, after which the validity of the relations is checked. If both of these relations are satisfied, then, by virtue of the theorem converse to Vieta's theorem, it is concluded that these numbers are the roots of the equation. If at least one of the relations is not satisfied, then these numbers are not the roots of the quadratic equation. This approach can be used when solving quadratic equations to check the found roots.

Example.

Which of the pairs of numbers 1) x 1 =−5, x 2 =3, or 2), or 3) is a pair of roots of the quadratic equation 4 x 2 −16 x+9=0?

Solution.

The coefficients of the given quadratic equation 4 x 2 −16 x+9=0 are a=4 , b=−16 , c=9 . According to Vieta's theorem, the sum of the roots of the quadratic equation must be equal to −b/a, that is, 16/4=4, and the product of the roots must be equal to c/a, that is, 9/4.

Now let's calculate the sum and product of the numbers in each of the three given pairs, and compare them with the values just obtained.

In the first case, we have x 1 +x 2 =−5+3=−2 . The resulting value is different from 4, therefore, further verification can not be carried out, but by the theorem, the inverse of Vieta's theorem, we can immediately conclude that the first pair of numbers is not a pair of roots of a given quadratic equation.

Let's move on to the second case. Here, that is, the first condition is satisfied. We check the second condition: , the resulting value is different from 9/4 . Therefore, the second pair of numbers is not a pair of roots of a quadratic equation.

The last case remains. Here and . Both conditions are met, so these numbers x 1 and x 2 are the roots of the given quadratic equation.

Answer:

The theorem, the reverse of Vieta's theorem, can be used in practice to select the roots of a quadratic equation. Usually, integer roots of the given quadratic equations with integer coefficients are selected, since in other cases this is quite difficult to do. At the same time, they use the fact that if the sum of two numbers is equal to the second coefficient of the quadratic equation, taken with a minus sign, and the product of these numbers is equal to the free term, then these numbers are the roots of this quadratic equation. Let's deal with this with an example.

Let's take the quadratic equation x 2 −5 x+6=0 . For the numbers x 1 and x 2 to be the roots of this equation, two equalities x 1 +x 2 \u003d 5 and x 1 x 2 \u003d 6 must be satisfied. It remains to choose such numbers. In this case, this is quite simple to do: such numbers are 2 and 3, since 2+3=5 and 2 3=6 . Thus, 2 and 3 are the roots of this quadratic equation.

The theorem converse to Vieta's theorem is especially convenient for finding the second root of the reduced quadratic equation when one of the roots is already known or obvious. In this case, the second root is found from any of the relations.

For example, let's take the quadratic equation 512 x 2 −509 x−3=0 . Here it is easy to see that the unit is the root of the equation, since the sum of the coefficients of this quadratic equation is zero. So x 1 =1 . The second root x 2 can be found, for example, from the relation x 1 x 2 =c/a. We have 1 x 2 =−3/512 , whence x 2 =−3/512 . So we have defined both roots of the quadratic equation: 1 and −3/512.

It is clear that the selection of roots is expedient only in the simplest cases. In other cases, to find the roots, you can apply the formulas of the roots of the quadratic equation through the discriminant.

Another practical application of the theorem, the inverse of Vieta's theorem, is the compilation of quadratic equations for given roots x 1 and x 2. To do this, it is enough to calculate the sum of the roots, which gives the coefficient of x with the opposite sign of the given quadratic equation, and the product of the roots, which gives the free term.

Example.

Write a quadratic equation whose roots are the numbers −11 and 23.

Solution.

Denote x 1 =−11 and x 2 =23 . We calculate the sum and product of these numbers: x 1 + x 2 \u003d 12 and x 1 x 2 \u003d −253. Therefore, these numbers are the roots of the given quadratic equation with the second coefficient -12 and the free term -253. That is, x 2 −12·x−253=0 is the desired equation.

Answer:

x 2 −12 x−253=0 .

Vieta's theorem is very often used in solving tasks related to the signs of the roots of quadratic equations. How is Vieta's theorem related to the signs of the roots of the reduced quadratic equation x 2 +p x+q=0 ? Here are two relevant statements:

If the intercept q is a positive number and if the quadratic equation has real roots, then either they are both positive or both are negative.
If the free term q is a negative number and if the quadratic equation has real roots, then their signs are different, in other words, one root is positive and the other is negative.

These statements follow from the formula x 1 x 2 =q, as well as the rules for multiplying positive, negative numbers and numbers with different signs. Consider examples of their application.

Example.

R is positive. According to the discriminant formula, we find D=(r+2) 2 −4 1 (r−1)= r 2 +4 r+4−4 r+4=r 2 +8 , the value of the expression r 2 +8 is positive for any real r , thus D>0 for any real r . Therefore, the original quadratic equation has two roots for any real values of the parameter r.

Now let's find out when the roots have different signs. If the signs of the roots are different, then their product is negative, and by the Vieta theorem, the product of the roots of the given quadratic equation is equal to the free term. Therefore, we are interested in those values of r for which the free term r−1 is negative. Thus, in order to find the values of r that are of interest to us, we need to solve a linear inequality r−1<0 , откуда находим r<1 .

Answer:

at r<1 .

Vieta formulas

Above, we talked about Vieta's theorem for a quadratic equation and analyzed the relations it asserts. But there are formulas that connect the real roots and coefficients not only of quadratic equations, but also of cubic equations, quadruple equations, and in general, algebraic equations degree n. They are called Vieta formulas.

We write the Vieta formulas for an algebraic equation of degree n of the form, while we assume that it has n real roots x 1, x 2, ..., x n (among them there may be the same):

Get Vieta formulas allows polynomial factorization theorem, as well as the definition of equal polynomials through the equality of all their corresponding coefficients. So the polynomial and its expansion into linear factors of the form are equal. Opening the brackets in the last product and equating the corresponding coefficients, we obtain the Vieta formulas.

In particular, for n=2 we have already familiar Vieta formulas for the quadratic equation .

For a cubic equation, the Vieta formulas have the form

It only remains to note that on the left side of the Vieta formulas there are the so-called elementary symmetric polynomials.

Bibliography.

Algebra: textbook for 8 cells. general education institutions / [Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, S. B. Suvorova]; ed. S. A. Telyakovsky. - 16th ed. - M. : Education, 2008. - 271 p. : ill. - ISBN 978-5-09-019243-9.
Mordkovich A. G. Algebra. 8th grade. At 2 pm Part 1. A textbook for students of educational institutions / A. G. Mordkovich. - 11th ed., erased. - M.: Mnemozina, 2009. - 215 p.: ill. ISBN 978-5-346-01155-2.
Algebra and the beginning of mathematical analysis. Grade 10: textbook. for general education institutions: basic and profile. levels / [Yu. M. Kolyagin, M. V. Tkacheva, N. E. Fedorova, M. I. Shabunin]; ed. A. B. Zhizhchenko. - 3rd ed. - M.: Enlightenment, 2010.- 368 p. : ill. - ISBN 978-5-09-022771-1.

Formulation and proof of Vieta's theorem for quadratic equations. Inverse Vieta theorem. Vieta's theorem for cubic equations and equations of arbitrary order.

Content

See also: The roots of a quadratic equation

Quadratic equations

Vieta's theorem

Let and denote the roots of the reduced quadratic equation
(1) .
Then the sum of the roots is equal to the coefficient at taken with the opposite sign. The product of the roots is equal to the free term:
;
.

A note about multiple roots

If the discriminant of equation (1) is zero, then this equation has one root. But, in order to avoid cumbersome formulations, it is generally accepted that in this case, equation (1) has two multiple, or equal, roots:
.

Proof one

Let us find the roots of equation (1). To do this, apply the formula for the roots of the quadratic equation:
;
;
.

Finding the sum of the roots:
.

To find the product, we apply the formula:
.
Then

.

The theorem has been proven.

Proof two

If the numbers and are the roots of the quadratic equation (1), then
.
We open the brackets.

.
Thus, equation (1) will take the form:
.
Comparing with (1) we find:
;
.

The theorem has been proven.

Inverse Vieta theorem

Let there be arbitrary numbers. Then and are the roots of the quadratic equation
,
where
(2) ;
(3) .

Proof of Vieta's converse theorem

Consider the quadratic equation
(1) .
We need to prove that if and , then and are the roots of equation (1).

Substitute (2) and (3) into (1):
.
We group the terms of the left side of the equation:
;
;
(4) .

Substitute in (4) :
;
.

Substitute in (4) :
;
.
The equation is fulfilled. That is, the number is the root of equation (1).

The theorem has been proven.

Vieta's theorem for the complete quadratic equation

Now consider the complete quadratic equation
(5) ,
where , and are some numbers. And .

We divide equation (5) by:
.
That is, we have obtained the above equation
,
where ; .

Then the Vieta theorem for the complete quadratic equation has the following form.

Let and denote the roots of the complete quadratic equation
.
Then the sum and product of the roots are determined by the formulas:
;
.

Vieta's theorem for a cubic equation

Similarly, we can establish connections between the roots of a cubic equation. Consider the cubic equation
(6) ,
where , , , are some numbers. And .
Let's divide this equation by:
(7) ,
where , , .
Let , , be the roots of equation (7) (and equation (6)). Then

.

Comparing with equation (7) we find:
;
;
.

Vieta's theorem for an nth degree equation

In the same way, you can find connections between the roots , , ... , , for the equation of the nth degree
.

Vieta's theorem for an nth degree equation has the following form:
;
;
;

.

To get these formulas, we write the equation in the following form:
.
Then we equate the coefficients at , , , ... , and compare the free term.

References:
I.N. Bronstein, K.A. Semendyaev, Handbook of Mathematics for Engineers and Students of Higher Educational Institutions, Lan, 2009.
CM. Nikolsky, M.K. Potapov et al., Algebra: a textbook for the 8th grade of educational institutions, Moscow, Education, 2006.