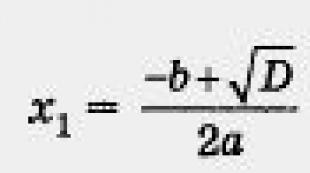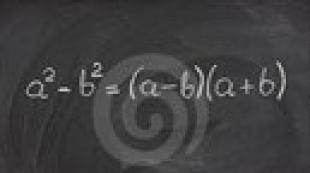Different ways to prove the Pythagorean theorem: examples, description and reviews. Independent problem solving
Class: 8
Lesson Objectives:
- Educational: achieve the assimilation of the Pythagorean theorem, instill the skills of calculating the unknown side of a right-angled triangle using two known ones, teach how to apply the Pythagorean theorem to solving simple problems
- Developing: contribute to the development of the ability to compare, observation, attention, the development of the ability to analytical and synthetic thinking, broadening one's horizons
- Educational: formation of the need for knowledge, interest in mathematics
Lesson type: new material presentation lesson
Equipment: computer, multimedia projector, presentation for the lesson ( Attachment 1)
Lesson plan:
- Organizing time
- oral exercises
- Research work, putting forward a hypothesis and testing it in particular cases
- Explanation of new material
a) About Pythagoras
b) Statement and proof of the theorem - Consolidation of the above through problem solving
- Homework, summarizing the lesson.
During the classes
Slide 2: Do the exercises
- Expand brackets: (3 + x) 2
- Calculate 3 2 + x 2 for x = 1, 2, 3, 4
– Is there a natural number whose square is 10, 13, 18, 25? - Find the area of a square with sides 11 cm, 50 cm, 7 dm.
What is the formula for the area of a square?
How to find the area of a right triangle?
Slide 3: Question answer
– An angle whose measure is 90°. (Straight)
The side opposite the right angle of the triangle. (Hypotenuse)
- Triangle, square, trapezium, circle - these are geometric ... (Shapes)
- The smaller side of a right triangle. (Katet)
- A figure formed by two rays emanating from one point. (Corner)
- A segment of a perpendicular drawn from the vertex of a triangle to the line containing the opposite side. (Height)
- A triangle with two equal sides . (Isosceles)
Slide 4: A task
Construct a right triangle with sides 3 cm, 4 cm and 6 cm.
The task is divided into rows.
| 1 row | 2 row | 3 row | |
| leg a | 3 | 3 | |
| leg b | 4 | 4 | |
| Hypotenuse With | 6 | 6 |
Questions:
- Did anyone get a triangle with given sides?
- What can be the conclusion? (A right triangle cannot be arbitrarily defined. There is a dependency between its sides).
- Measure the resulting sides. ( The approximate average result from each row is entered in the table)
| 1 row | 2 row | 3 row | |
| leg a | 3 | 3 | ~4,5 |
| leg b | 4 | ~5,2 | 4 |
| Hypotenuse With | ~5 | 6 | 6 |
- Try to establish a relationship between the legs and the hypotenuse in each of the cases.
(It is proposed to recall oral exercises and check the same relationship between other numbers).
- Attention is drawn to the fact that the exact result will not work, because. measurements cannot be considered accurate.
The teacher asks for guesses (hypotheses): students formulate.
- Yes, indeed, there is a relationship between the hypotenuse and the legs, and the first to prove it was the scientist, whose name you will name yourself. This theorem is named after him.
Slide 5: Decipher
Slide 6: Pythagoras of Samos
Who will name the topic of today's lesson?
Students in notebooks write down the topic of the lesson: “The Pythagorean Theorem”
The Pythagorean theorem is one of the main theorems of geometry. With its help, many other theorems are proved and problems from various fields are solved: physics, astronomy, construction, etc. It was known long before Pythagoras proved it. The ancient Egyptians used it when building a right triangle with sides of 3, 4 and 5 units using a rope to build right angles when laying buildings, pyramids. Therefore, such a triangle is called Egyptian triangle.
There are over three hundred ways to prove this theorem. We will look at one of them today.
Slide 7: Pythagorean theorem
Theorem: In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs.
Given:
Right triangle,
a, b - legs, With- hypotenuse
Prove:
Proof.
1. We continue the legs of a right triangle: leg a- for length b, leg b- for length a.
What shape can a triangle be built to? Why up to a square? What will be the side of the square?
2. We complete the triangle to a square with a side a + b.
How can you find the area of this square?
3. The area of the square is
- Let's break the square into parts: 4 triangles and a square with side c.
How else can you find the area of the original square?
Why are the resulting right triangles congruent?
4. On the other hand,
5. Equate the resulting equalities:
![]()
The theorem has been proven.
There is a comic formulation of this theorem: “Pythagorean pants are equal in all directions.” Probably, such a formulation is due to the fact that this theorem was originally established for an isosceles right triangle. Moreover, it sounded a little different: “The area of a square built on the hypotenuse of a right triangle is equal to the sum of the areas of squares built on its legs.”
Slide 8: Another formulation of the Pythagorean theorem
And I will give you another formulation of this theorem in verse:
If we are given a triangle
And, moreover, with a right angle,
That is the square of the hypotenuse
We can always easily find:
We build the legs in a square,
We find the sum of degrees
And in such a simple way
We will come to the result.
- So, today you got acquainted with the most famous theorem of planimetry - the Pythagorean theorem. How is the Pythagorean theorem formulated? How else can it be formulated?
Primary fixation of the material
Slide 9: Solution of problems according to ready-made drawings.
Slide 10: Solving problems in a notebook
Three students are called to the board at the same time to solve problems.
Slide 11: Problem of the 12th century Indian mathematician Bhaskara
Summing up the lesson:
What new did you learn at the lesson today?
- Formulate the Pythagorean theorem.
- What did you learn to do in the lesson?
Homework:
– Learn the Pythagorean theorem with proof
- Tasks from textbook No. 483 c, d; No. 484 in, city of
– For more advanced students: find other proofs of the Pythagorean theorem, learn one of them.
The work of the class as a whole is evaluated, highlighting individual students.
Lesson on the topic: "The Pythagorean Theorem"
Type of lesson: lesson learning new material. (according to the textbook “Geometry, 7–9”, a textbook for educational institutions; L.S. Atanasyan et al. - 12th ed. - M .: Education, 2009).
Target:
introduce students to the Pythagorean theorem and historical information related to this theorem; develop interest in the study of mathematics, logical thinking; Attention.
During the classes:
1. Organizational moment.
SLIDE 2 Fairy tale "House".
The topic of our lesson is "The Pythagorean Theorem". Today in the lesson we will get acquainted with the biography of Pythagoras, we will study one of the most famous geometric theorems of antiquity, called the Pythagorean theorem, one of the main theorems of planimetry.
2. Actualization of knowledge.(Preparation for the study of new material, the material that will be needed in the proof of the theorem is repeated)
1) Questions:
What quadrilateral is called a square?
How to find the area of a square?
Which triangle is called a right triangle?
What are the sides of a right triangle called?
How to find the area of a right triangle?
3. Learning new material.
1) History reference.
SLIDE 3 and 4.
The great scientist Pythagoras was born around 570 BC. on the island of Samos. Pythagoras' father was Mnesarchus, a gem-carver. The name of Pythagoras' mother is unknown. According to many ancient testimonies, the born boy was fabulously handsome, and soon showed his outstanding abilities. Like any father, Mnesarchus dreamed that his son would continue his work - the craft of a goldsmith. Life judged otherwise. The future great mathematician and philosopher already in childhood showed great abilities for the sciences.
Pythagoras is credited with studying the properties of integers and proportions, proving the Pythagorean theorem, etc. Pythagoras is not a name, but a nickname that the philosopher received for always speaking correctly and convincingly, like a Greek oracle. (Pythagoras - "persuasive speech".)
With his speeches, he acquired 2,000 students, who, together with their families, formed a school-state, where the laws and rules of Pythagoras were in force. The school of Pythagoras, or, as it is also called, the Pythagorean Union, was at the same time a philosophical school, a political party, and a religious brotherhood.
The favorite geometric figure of the Pythagoreans was the pentagram, also called the Pythagorean star. The Pythagoreans used this figure, drawing it in the sand, to greet and recognize each other. The pentagram served as their password and was a symbol of health and happiness.
Tradition says that when Pythagoras came to the theorem that bears his name, he brought 100 bulls to the gods. In the 500 BC, Pythagoras was killed in a street fight during a popular uprising. Currently, there are about 200 proofs of the Pythagorean theorem.
Statement of the theorem
2) Proof of the theorem.
Let's build a rectangle to a square with side a + b.
The children, with the help of a teacher, prove the theorem according to the drawing, then write the proof in a notebook.
Proof:
square area


 - the theorem is proved.
- the theorem is proved.
4. Primary consolidation of knowledge.
Textbook work (Application of the Pythagorean theorem to problem solving).
Problems are solved on the board and in notebooks.
Conclusion: using the Pythagorean theorem, you can solve two types of problems:
1. Find the hypotenuse of a right triangle if the legs are known.

2. Find the leg if the hypotenuse and the other leg are known.

 .
.
5. Independent problem solving.
No. 483 (b), 484 (b)
6. Homework: P 54, No. 483 (d), 484 (d).
7. The result of the lesson.
What new did you learn at the lesson today?
For which triangles does the Pythagorean theorem apply?
Finish the lesson with a poem.
Many people know Chamisso's sonnet:
Truth will remain eternal, how soon
A weak person will know it!
And now the Pythagorean theorem
Verna, as in his distant age.
The sacrifice was plentiful
Gods from Pythagoras. One hundred bulls
He gave to the slaughter and burning
Behind the light is a ray that came from the clouds.
Therefore, ever since
A little truth is born into the world,
The bulls roar, sensing her, following.
They can't stop the light
And can only close their eyes to tremble
From the fear that Pythagoras instilled in them.

Question - answer Angle whose measure is 90 ° DIRECT The side lying opposite the right angle of the triangle HYPOTENUSE Triangle, square, trapezoid, circle are geometric ... FIGURES The smaller side of a right triangle CATETH The figure formed by two rays emanating from one point ANGLE Perpendicular segment drawn from the vertex of a triangle to the line containing the opposite side HEIGHT A triangle whose two sides are equal isosceles


Pythagoras of Samos (c. 580 - c. 500 BC) Ancient Greek mathematician and philosopher. Born on the island of Samos. He organized his own school - the school of Pythagoras (Pythagorean Union), which was at the same time a philosophical school, a political party, and a religious brotherhood. He was the first to prove the relationship between the hypotenuse and the legs of a right triangle.





Problem of the Indian mathematician of the XII century Bhaskara On the bank of the river a lonely poplar grew. Suddenly a gust of wind broke its trunk. The poor poplar has fallen. And the angle of a straight line With the course of the river, its trunk was. Remember now that in this place the river B was only four feet wide. The head leaned at the edge of the river. There are only three feet left from the trunk, I beg you, tell me soon now: How high is the poplar tree?

Shapovalova L.A. (station Egorlykskaya, MBOU ESOSH No. 11)
1. Glazer G.I. History of mathematics at school VII - VIII grades, a guide for teachers, - M: Education, 1982.
2. Dempan I.Ya., Vilenkin N.Ya. "Behind the pages of a mathematics textbook" Handbook for students in grades 5-6. – M.: Enlightenment, 1989.
3. Zenkevich I.G. "Aesthetics of the Mathematics Lesson". – M.: Enlightenment, 1981.
4. Litzman V. The Pythagorean theorem. - M., 1960.
5. Voloshinov A.V. "Pythagoras". - M., 1993.
6. Pichurin L.F. "Beyond the Pages of an Algebra Textbook". - M., 1990.
7. Zemlyakov A.N. "Geometry in the 10th grade." - M., 1986.
8. Newspaper "Mathematics" 17/1996.
9. Newspaper "Mathematics" 3/1997.
10. Antonov N.P., Vygodskii M.Ya., Nikitin V.V., Sankin A.I. "Collection of Problems in Elementary Mathematics". - M., 1963.
11. Dorofeev G.V., Potapov M.K., Rozov N.Kh. "Mathematics Handbook". - M., 1973.
12. Shchetnikov A.I. "The Pythagorean doctrine of number and magnitude". - Novosibirsk, 1997.
13. “Real numbers. Irrational expressions» Grade 8. Tomsk University Press. – Tomsk, 1997.
14. Atanasyan M.S. "Geometry" grade 7-9. – M.: Enlightenment, 1991.
15. URL: www.moypifagor.narod.ru/
16. URL: http://www.zaitseva-irina.ru/html/f1103454849.html.
This academic year, I got acquainted with an interesting theorem, known, as it turned out, from ancient times:
"The square built on the hypotenuse of a right triangle is equal to the sum of the squares built on the legs."
Usually the discovery of this statement is attributed to the ancient Greek philosopher and mathematician Pythagoras (VI century BC). But the study of ancient manuscripts showed that this statement was known long before the birth of Pythagoras.
I wondered why, in this case, it is associated with the name of Pythagoras.
Relevance of the topic: The Pythagorean theorem is of great importance: it is used in geometry literally at every step. I believe that the works of Pythagoras are still relevant, because wherever we look, everywhere we can see the fruits of his great ideas, embodied in various branches of modern life.
The purpose of my research was: to find out who Pythagoras was, and what relation he has to this theorem.
Studying the history of the theorem, I decided to find out:
Are there other proofs of this theorem?
What is the significance of this theorem in people's lives?
What role did Pythagoras play in the development of mathematics?
From the biography of Pythagoras
Pythagoras of Samos is a great Greek scientist. Its fame is associated with the name of the Pythagorean theorem. Although now we already know that this theorem was known in ancient Babylon 1200 years before Pythagoras, and in Egypt 2000 years before him a right-angled triangle with sides 3, 4, 5 was known, we still call it by the name of this ancient scientist.
Almost nothing is known for certain about the life of Pythagoras, but a large number of legends are associated with his name.
Pythagoras was born in 570 BC on the island of Samos.
Pythagoras had a handsome appearance, wore a long beard, and a golden diadem on his head. Pythagoras is not a name, but a nickname that the philosopher received for always speaking correctly and convincingly, like a Greek oracle. (Pythagoras - "persuasive speech").
In 550 BC, Pythagoras makes a decision and goes to Egypt. So, an unknown country and an unknown culture opens up before Pythagoras. Much amazed and surprised Pythagoras in this country, and after some observations of the life of the Egyptians, Pythagoras realized that the path to knowledge, protected by the caste of priests, lies through religion.

After eleven years of study in Egypt, Pythagoras goes to his homeland, where along the way he falls into Babylonian captivity. There he gets acquainted with the Babylonian science, which was more developed than the Egyptian. The Babylonians knew how to solve linear, quadratic and some types of cubic equations. Having escaped from captivity, he could not stay long in his homeland because of the atmosphere of violence and tyranny that reigned there. He decided to move to Croton (a Greek colony in northern Italy).

It is in Croton that the most glorious period in the life of Pythagoras begins. There he established something like a religious-ethical brotherhood or a secret monastic order, whose members were obliged to lead the so-called Pythagorean way of life.
Pythagoras and the Pythagoreans
Pythagoras organized in a Greek colony in the south of the Apennine Peninsula a religious and ethical brotherhood, such as a monastic order, which would later be called the Pythagorean Union. The members of the union had to adhere to certain principles: firstly, to strive for the beautiful and glorious, secondly, to be useful, and thirdly, to strive for high pleasure.
The system of moral and ethical rules, bequeathed by Pythagoras to his students, was compiled into a kind of moral code of the Pythagoreans "Golden Verses", which were very popular in the era of Antiquity, the Middle Ages and the Renaissance.
The Pythagorean system of studies consisted of three sections:
Teachings about numbers - arithmetic,
Teachings about figures - geometry,
Teachings about the structure of the universe - astronomy.
The education system laid down by Pythagoras lasted for many centuries.
The school of Pythagoras did much to give geometry the character of a science. The main feature of the Pythagorean method was the combination of geometry with arithmetic.

Pythagoras dealt a lot with proportions and progressions and, probably, with the similarity of figures, since he is credited with solving the problem: “Construct a third one, equal in size to one of the data and similar to the second, based on the given two figures.”
Pythagoras and his students introduced the concept of polygonal, friendly, perfect numbers and studied their properties. Arithmetic, as a practice of calculation, did not interest Pythagoras, and he proudly declared that he "put arithmetic above the interests of the merchant."
Members of the Pythagorean Union were residents of many cities in Greece.
The Pythagoreans also accepted women into their society. The Union flourished for more than twenty years, and then the persecution of its members began, many of the students were killed.
There were many different legends about the death of Pythagoras himself. But the teachings of Pythagoras and his disciples continued to live.
From the history of the creation of the Pythagorean theorem
It is currently known that this theorem was not discovered by Pythagoras. However, some believe that it was Pythagoras who first gave its full proof, while others deny him this merit. Some attribute to Pythagoras the proof which Euclid gives in the first book of his Elements. On the other hand, Proclus claims that the proof in the Elements is due to Euclid himself. As we can see, the history of mathematics has almost no reliable concrete data on the life of Pythagoras and his mathematical activity.

Let's start our historical review of the Pythagorean theorem with ancient China. Here the mathematical book of Chu-pei attracts special attention. This essay says this about the Pythagorean triangle with sides 3, 4 and 5:
"If a right angle is decomposed into its component parts, then the line connecting the ends of its sides will be 5 when the base is 3 and the height is 4."
It is very easy to reproduce their method of construction. Take a rope 12 m long and tie it to it along a colored strip at a distance of 3 m. from one end and 4 meters from the other. A right angle will be enclosed between sides 3 and 4 meters long.
Geometry among the Hindus was closely connected with the cult. It is highly probable that the hypotenuse squared theorem was already known in India around the 8th century BC. Along with purely ritual prescriptions, there are works of a geometrically theological nature. In these writings, dating back to the 4th or 5th century BC, we meet with the construction of a right angle using a triangle with sides 15, 36, 39.
In the Middle Ages, the Pythagorean theorem defined the limit, if not of the greatest possible, then at least of good mathematical knowledge. The characteristic drawing of the Pythagorean theorem, which is now sometimes turned by schoolchildren, for example, into a top hat dressed in a robe of a professor or a man, was often used in those days as a symbol of mathematics.

In conclusion, we present various formulations of the Pythagorean theorem translated from Greek, Latin and German.
Euclid's theorem reads (literal translation):
"In a right triangle, the square of the side spanning the right angle is equal to the squares on the sides that enclose the right angle."

As you can see, in different countries and different languages there are different versions of the formulation of the familiar theorem. Created at different times and in different languages, they reflect the essence of one mathematical pattern, the proof of which also has several options.
Five Ways to Prove the Pythagorean Theorem
ancient chinese evidence
In an ancient Chinese drawing, four equal right-angled triangles with legs a, b and hypotenuse c are stacked so that their outer contour forms a square with side a + b, and the inner one forms a square with side c, built on the hypotenuse
a2 + 2ab + b2 = c2 + 2ab

Proof by J. Gardfield (1882)
Let us arrange two equal right-angled triangles so that the leg of one of them is a continuation of the other.
The area of the trapezoid under consideration is found as the product of half the sum of the bases and the height

On the other hand, the area of the trapezoid is equal to the sum of the areas of the obtained triangles:
Equating these expressions, we get:
The proof is simple
This proof is obtained in the simplest case of an isosceles right triangle.
Probably, the theorem began with him.
Indeed, it is enough just to look at the tiling of isosceles right triangles to see that the theorem is true.
For example, for the triangle ABC: the square built on the hypotenuse AC contains 4 initial triangles, and the squares built on the legs contain two. The theorem has been proven.
Proof of the ancient Hindus
A square with a side (a + b), can be divided into parts either as in fig. 12. a, or as in fig. 12b. It is clear that parts 1, 2, 3, 4 are the same in both figures. And if equals are subtracted from equals (areas), then equals will remain, i.e. c2 = a2 + b2.

Euclid's proof
For two millennia, the most common was the proof of the Pythagorean theorem, invented by Euclid. It is placed in his famous book "Beginnings".
Euclid lowered the height BH from the vertex of the right angle to the hypotenuse and proved that its extension divides the square completed on the hypotenuse into two rectangles, the areas of which are equal to the areas of the corresponding squares built on the legs.

The drawing used in the proof of this theorem is jokingly called "Pythagorean pants". For a long time he was considered one of the symbols of mathematical science.
Application of the Pythagorean theorem
The significance of the Pythagorean theorem lies in the fact that most of the theorems of geometry can be derived from it or with its help and many problems can be solved. In addition, the practical significance of the Pythagorean theorem and its inverse theorem is that they can be used to find the lengths of segments without measuring the segments themselves. This, as it were, opens the way from a straight line to a plane, from a plane to volumetric space and beyond. It is for this reason that the Pythagorean theorem is so important for humanity, which seeks to discover more dimensions and create technologies in these dimensions.
Conclusion
The Pythagorean theorem is so famous that it is difficult to imagine a person who has not heard about it. I learned that there are several ways to prove the Pythagorean theorem. I studied a number of historical and mathematical sources, including information on the Internet, and realized that the Pythagorean theorem is interesting not only for its history, but also because it occupies an important place in life and science. This is evidenced by the various interpretations of the text of this theorem given by me in this paper and the ways of its proofs.
So, the Pythagorean theorem is one of the main and, one might say, the most important theorem of geometry. Its significance lies in the fact that most of the theorems of geometry can be deduced from it or with its help. The Pythagorean theorem is also remarkable in that in itself it is not at all obvious. For example, the properties of an isosceles triangle can be seen directly on the drawing. But no matter how much you look at a right triangle, you will never see that there is a simple relation between its sides: c2 = a2 + b2. Therefore, visualization is often used to prove it. The merit of Pythagoras was that he gave a full scientific proof of this theorem. The personality of the scientist himself, whose memory is not accidentally preserved by this theorem, is interesting. Pythagoras is a wonderful speaker, teacher and educator, the organizer of his school, focused on the harmony of music and numbers, goodness and justice, knowledge and a healthy lifestyle. He may well serve as an example for us, distant descendants.
Bibliographic link
Tumanova S.V. SEVERAL WAYS TO PROVE THE PYTHAGOREAN THEOREM // Start in science. - 2016. - No. 2. - P. 91-95;URL: http://science-start.ru/ru/article/view?id=44 (date of access: 01/10/2020).
Pythagorean theorem- one of the fundamental theorems of Euclidean geometry, establishing the relation
between the sides of a right triangle.
It is believed that it was proved by the Greek mathematician Pythagoras, after whom it is named.
Geometric formulation of the Pythagorean theorem.
The theorem was originally formulated as follows:
In a right triangle, the area of the square built on the hypotenuse is equal to the sum of the areas of the squares,
built on catheters.
Algebraic formulation of the Pythagorean theorem.
In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs.
That is, denoting the length of the hypotenuse of the triangle through c, and the lengths of the legs through a and b:
Both formulations pythagorean theorems are equivalent, but the second formulation is more elementary, it does not
requires the concept of area. That is, the second statement can be verified without knowing anything about the area and
by measuring only the lengths of the sides of a right triangle.
The inverse Pythagorean theorem.
If the square of one side of a triangle is equal to the sum of the squares of the other two sides, then
triangle is rectangular.
Or, in other words:
For any triple of positive numbers a, b and c, such that
there is a right triangle with legs a and b and hypotenuse c.
The Pythagorean theorem for an isosceles triangle.

Pythagorean theorem for an equilateral triangle.

Proofs of the Pythagorean theorem.
At the moment, 367 proofs of this theorem have been recorded in the scientific literature. Probably the theorem
Pythagoras is the only theorem with such an impressive number of proofs. Such diversity
can only be explained by the fundamental significance of the theorem for geometry.
Of course, conceptually, all of them can be divided into a small number of classes. The most famous of them:
proof of area method, axiomatic and exotic evidence(for example,
by using differential equations).
1. Proof of the Pythagorean theorem in terms of similar triangles.
The following proof of the algebraic formulation is the simplest of the proofs constructed
directly from the axioms. In particular, it does not use the concept of the area of a figure.
Let ABC there is a right angled triangle C. Let's draw a height from C and denote
its foundation through H.
Triangle ACH similar to a triangle AB C on two corners. Likewise, the triangle CBH similar ABC.
By introducing the notation:

we get:
![]() ,
,
which matches -
Having folded a 2 and b 2 , we get:
or , which was to be proved.
2. Proof of the Pythagorean theorem by the area method.
The following proofs, despite their apparent simplicity, are not so simple at all. All of them
use the properties of the area, the proof of which is more complicated than the proof of the Pythagorean theorem itself.
- Proof through equicomplementation.
 Arrange four equal rectangular
Arrange four equal rectangular
triangle as shown in the picture
on right.
Quadrilateral with sides c- square,
since the sum of two acute angles is 90°, and
the developed angle is 180°.
The area of the whole figure is, on the one hand,
area of a square with side ( a+b), and on the other hand, the sum of the areas of four triangles and
![]()
![]()
Q.E.D.
3. Proof of the Pythagorean theorem by the infinitesimal method.

Considering the drawing shown in the figure, and
watching the side changea, we can
write the following relation for infinite
small side incrementsWith and a(using similarity
triangles):
Using the method of separation of variables, we find:
A more general expression for changing the hypotenuse in the case of increments of both legs:
Integrating this equation and using the initial conditions, we obtain:
Thus, we arrive at the desired answer:
As it is easy to see, the quadratic dependence in the final formula appears due to the linear
proportionality between the sides of the triangle and the increments, while the sum is related to the independent
contributions from the increment of different legs.
A simpler proof can be obtained if we assume that one of the legs does not experience an increment
(in this case, the leg b). Then for the integration constant we get: